Series 4, Year 18

One winter afternoon the soviet generals were out of patience while watching imperialistic West and pressed The Red Button to fire nuclear bomb. Immediately after that the young lieutenant entered the room admitting the error in calculation of the rocket's trajectory. Instead to the New York the racket was aiming to the friendly Cuba.

Luckily enough, another rocket is ready and can be sent to shoot down the first one and avoid disturbance in between socialistic countries. The original rocket was fired at the speed $v$ under the angle $α$. What angle $β$ should be set for the second rocket to shoot down the first rocket if the time delay between the launches is $T$.

Discuss when the peace between socialistic countries can be saved and when not. And of course everyone knows, that the Earth is flat and the gravitation field is homogeneous.

A small cylinder of radius $r$ and mass $m$ is rolling on the inclined plane. At the end of the plane it follows smoothly to horizontal motion and starts to wind onto itself string of the linear density $ρ$. At what distance from the end of inclined plane will the cylinder stop? You know the height of the inclined plane $h$ and it slope $α$. The friction is negligible.

One of the winners of Superstar (equivalent of 'You are a star'/'Eurovision song contest') has suddenly a problem. His new limousine is too long to fit in his old shed. His friend, student of physics and lover of Albert Einstein work, suggested that if the limousine drives fast it contracts in the frame of stationary observer. And if the speed is large enough, it will fit into the shed.

At the beginning and the end of shed are trap doors which fall when the limousine is all inside. But from the point of view of driver the in the limousine, due to the length contraction the shed is shorter and the limousine cannot fit in!

Decide, if is possible to park the limousine in the shed.

The frequency of photon emitted by the nucleus of radioactive iron is not always the same, but is slightly different (it is true also for other elements). To make thinks simpler, assume that the energy of photon in the frame connected with the resting nucleus of iron is randomly in interval ( $E_{0}-ΔE,E_{0}+ΔE)$, where $E_{0}=14,4\;\mathrm{keV}$ (keV = kiloelektronVolt), $ΔE≈10^{-8}\;\mathrm{eV}$ (1 eV = 1,602 \cdot 10^{-19} J ).

• When the photon is emitted from a stationary nucleus the nucleus acquires opposite momentum to the photon. Calculate kinetic energy of the atom and compare it with the $ΔE$.
• So called Mössbauer effect is the transfer of momentum of recoil to the crystal (whose the atom is part of). Calculate kinetic energy of the crystal (the shift in photon energy) assuming that the crystal consist of 10^{23} atoms.

Same as the emission of photon also excitation occurs. The photon can be absorbed only if its energy in the rest frame of atom is in interval ( $E_{0}-ΔE,E_{0}+ΔE)$.

• Decide if the resting atom of iron can absorb photon emitted by another resting atom of iron.
• Calculate the relative speed of two pieces of iron needed for Doppler effect to forbid the absorption of the photon in the second piece of iron. The Doppler effect is the change in the frequency $f$, of the photon when the source is coming closer to the observer at the speed $v$. The frequency is changed to

$f′=(1+v⁄c)f$.

Assume that the Mössbauer effect takes place at the emission.

Find all the needed constants in tables.

Having a small thin-wall glass and circling with the wetted finger on its edge very loud sound can be produced. When the water is poured into the glass, the pitch (frequency) of the sound is decreasing with increasing water level. Try this experimentally and explain this effect.

The FYKOS organisers had a long discussion over a cup of tea. The point was the cooling of the tea. Whether the tea cup is cooled by evaporation, conduction of heat or radiation of heat. Try to solve the same problem using some experiment.

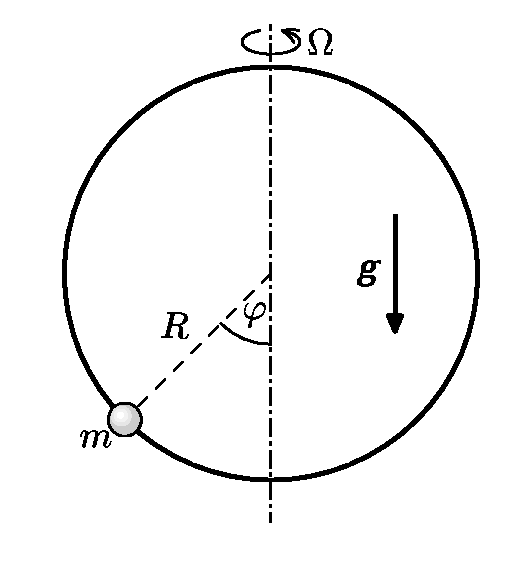
The small bead of the mass $m$ is sliding without friction on the wire loop of the shape of circle of radius $R$, the loop is rotating with the constant angular speed $Ω$ around the horizontal axis (see image).

• Select appropriate generalised coordinate and construct Lagrange function of the problem.
• Construct Lagrange equation of the 2nd order which describes the motion of the bead.
• Decide, when the equilibrium position in the lowest point of the loop stable and unstable, depending on $Ω$. For $Ω$, when this position is stable calculate the period of the oscillations of the bead around this stable position.
• For a bonus point find next stable equilibrium positions and discuss if they are stable or unstable. For stable equilibrium positions calculate frequencies of oscillations.