Search

A very large piece of ice (let us say with diameter 1 km) is placed near a Sun-like star to a circular orbit. It is placed so close, that the equillibrium temperature of a black body at this distance would be approximately 30 ° C. What will happen with such an asteroid and its orbit? The asteroid is not tidally locked.

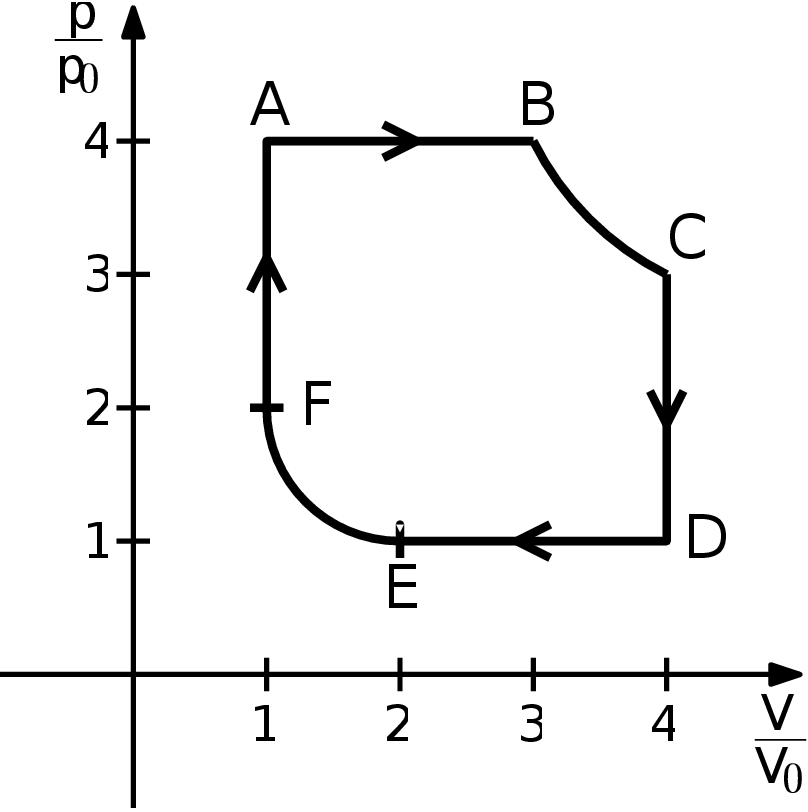
Consider a heat engine filled with a diatomic gas. This engine works thanks to a cycle ABCDEFA as shown in the picture. The 6 processes that make up the cycle are

• A $→$ B - isobaric heating from a state 4$p_{0}$ and $V_{0}$ (let us denote temperature at A 4$T_{0})$ to a state with volume 3$V_{0}$,
• B $→$ C - isothermic expansion to volume 4$V_{0}$,
• C $→$ D - isochoric cooling to pressure $p_{0}$,
• D $→$ E - isobaric cooling to volume 2$V_{0}$,
• E $→$ F - isothermic compression to volume $V_{0}$,
• F $→$ A - isochoric heating to pressure 4$p_{0}$. Determine the remaining state variables in B, C, D, E, and F, the maximal and the minimal temperature of the ideal gas during the process (as a multiple of $T_{0})$, heat received and lost by the gas in each process, and the overall efficiency of the engine. Compare this efficiency with that of a Carnot engine working between the same minimal and maximal temperatures. Assume for simplicity that the molar amount of the gas does not change and there are no chemical changes during the cycle. A sketch can be seen in figure.

Bonus: Do the same for a much simpler „square“ cycle, ABCDA, where the gas starts in a state $p_{0}$, $V_{0}$ and $T_{0}$ and izochorically heats up to 4$p_{0}$, isobarically heats up and expands to 4$V_{0}$, isochorically cools down to $p_{0}$ and isobarically cools down to $V_{0}$. Compare the efficiency of these two heat engines and suggest which one is better.

Many types of materials, mostly metals, have increasing dependence of resistivity on temperature. However, there are semiconductors or graphite which show a decreasing dependence. And you have also probably heard about superconductivity, the natural phenomenon when a cooled material shows almost no electrical resistance and becomes a perfect conductor. Our current state of knowledge says that the temperature of a superconductor must be well below room temperature, but let's assume that the equation defining the resistance is $R=$ R_{0} (1 + αΔt)$$, where $R_{0}is$ the resistance at room temperature, $αis$ the temperature coefficient of resistance and $Δt$ is the temperature difference with respect to room temperature, and the equation holds for any temperature. Using this equation and coefficients $α_{C}=-0.5\cdot 10^{-3}K^{-1}$ for graphite and $α_{Si}=-75\cdot 10^{-3}K^{-1}$ for silicon, we obtain zero resistance for high temperatures. Determine these two temperatures and explain why the superconducting phenomenon does not work this way, i.e. neither carbon nor silicon are superconductors at high temperatures.

Guns can be considered to be heat engines. Calculate the efficiency of a gun, say a pistol or a rifle.

Three substances: water, steel and rum are put in a pot, which effectively doesn't convect any heat. The water has mass $m_{v}=0,5\;\mathrm{kg}$, temperature $t_{v}=90°F$ and specific heat capacity $c_{v}=1kcal\cdot \;\mathrm{kg}^{-1}\cdot K^{-1}$. The steel is in form of a cylinder, that has mass $m_{o}=200g$, temperature $t_{o}=60\;\mathrm{°C}$ and specific heat capacity $c_{o}=0,260kJ\cdot \;\mathrm{kg}^{-1}\cdot °F^{-1}$. Rum has mass $m_{r}=100000mg$, temperature $t_{r}=270K$ and specific heat capacity $c_{r}=3,5J\cdot g^{-1}\cdot \;\mathrm{°C}^{-1}$. What will be the temperature (in degrees centigrade) of the system when it reaches balance?

Neutral particle beams are used in various fusion devices to heat up plasma. In a device like that, ions of deuterium are accelerated to high energy before they are neutralized, keeping almost the initial speed. Particles coming out of the neutralizer of the COMPASS tokamak have energy 40 keV and the current in the beam just before the neutralization is 12 A. What is the force acting on the beam generator? What is its power?

• Find, in literature or online, the change of enthalpy and Gibbs free energy in the following reaction

$$2\,\;\mathrm{H}_2 \mathrm{O}_2\longrightarrow2\,\mathrm{H}_2\mathrm{O},$$

where both the reactants and the product are gases at standard conditions. Find the change of entropy in this reaction. Give results per mole.

• Power flux in a photon gas is given by

$j=\frac{3}{4}\frac{k_\;\mathrm{B}^4\pi^2}{45\hbar^3c^3}cT^4$.

Substitute the values of the constants and compare the result with the Stefan-Boltzmann law.

• Calculate the internal energy and the Gibbs free energy of a photon gas. Use the internal energy to write the temperature of a photon gas as a function of its volume for an adiabatic expansion (a process with $δQ=0)$.

Hint: The law for an adiabatic process with an ideal gas was derived in the second part of this series (Czech only).

• Considering a photon gas, show that if $δQ⁄T$ is given by

$$\delta Q / T = f_{,T} \;\mathrm{d} T f_{,V} \mathrm{d} V\,,$$

then functions $f_{,T}$ and $f_{,V}$ obey the necessary condition for the existence of entropy, that is

$$\frac{\partial f_{,T}(T, V)}{\partial V} = \frac{\partial f_{,V}(T, V)}{\partial T} $$

As we all know, it is always a little bit chilly in the caves of central Europe, usually about 4 °C. Why, on the other hand, is it always warm in the underground (subway, metro) throughout the whole year? Is more heat produced by the people present or by the technology?

• Use the relation for entropy of ideal gas from the solution of third serial problem

$$S(U, V, N) = \frac{s}{2}n R \ln \left( \frac{U V^{{\kappa} -1}}{\frac{s}{2}R n^{\kappa} } \right) nR s_0$$

and the relation for the change of the entropy

$$\;\mathrm{d} S = \frac{1}{T}\mathrm{d} U \frac{p}{T} \mathrm{d} V - \frac{\mu}{T} \mathrm{d} N$$

to calculate chemical potential as a function of $U$, $VaN$. Modify it further to get the function of $T$, $pandN$.

Hint: The coefficients like 1 ⁄ $T$ in front of d$U$ can be calculated as a partial derivative of $S(U,V,N)$ by $U$. Don't forget that ln$(a⁄b)=\lna-\lnb$ and that $n=N⁄N_{A}$.

Bonus: Express similarly temperature and pressure as functions of $U$, $VandN$. Eliminate the pressure dependence to get the equation of state.

• Is the chemical potential of an ideal gas positive or negative? (Assume $s_{0}$ is negligible.)?

• What will happen with a gas in a piston if the gas is connected to a reservoir of temperature $T_{r}?$ The piston can move freely and there is nothing acting on it from the other side. Describe what happens if we allow only quasistatic processes. How much work can we extract? Is it true that the free energy is minimized?

Hint: To calculate the work, this equation can be useful:

$$\int _{a}^{b} \frac{1}{x} \;\mathrm{d}x = \ln \frac{b}{a}.$$

• We defined the enthalpy as $H=U+pV$ and the Gibb's free energy as $G=U-TS+pV$. What are the natural variables of these two potentials? What other thermodynamic quantities do we obtain by differentiating these potentials by their most natural variables?

• Calculate the change of grandcanonic potential d$Ω$ from its definition $Ω=F-μN$.

Let's have a Kofola (a Czech soft drink) with the energetic value $Q_{k}=1360kJ⁄\;\mathrm{kg}$ and temperature $t_{k}=24\;\mathrm{°C}$ and another Kofola, this time sugar-free, with the energetic value $Q_{free}=14.4kJ⁄\;\mathrm{kg}$ and temperature $t_{free}=4\;\mathrm{°C}$. If we assume other behaviour and constants are very similar to water, what temperature would have a mixture of these two for which the total energy gain would be none.