Search

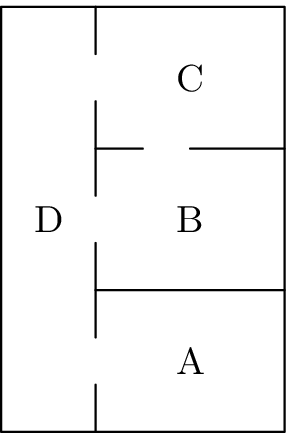
Mára decided to infect Aleš's four room apartment with pharaoh ants (top view of the apartment is on the picture). Ants are running all over the place but you can assume the following model of their motion. Every five minutes 60$%$ of ants in each room moves to the neighboring rooms and the rest stay where they are now. If there is more than one neighboring room assume that the same amount of ants moved to every one of them. This process repeats itself every five minutes (yes, assume only discrete time). The ants cannot move in or out of the apartment and they are immortal.

• If Mára places 1000 ants into the hallway (D) how many ants will be in each of the rooms after 5, 10 and 15 minutes? (2 body)

• If at some point we found out that the distribution of ants in the rooms is $N_{A}=12$, $N_{B}=25$, $N_{C}=25$ a$N_{D}=37$, how where they distributed 5 minutes earlier? (1 bod)

• *Bonus:** How would they be distributed after essentially infinite time if we start with 1000 ants in the hallway again? Does it matter how where they distributed in the beginning? And finally - will the distribution of ants in the rooms reach a stationary value or will it oscillate? (bonus points)

Mother is connected to a stroller of mass $m$ with a string of length $l$ that is initially fully stretched. The coefficient of friction between the floor and mother resp. stroller is $f$. Mother starts pulling the stroller with constant velocity $v$ that is perpendicular to the initial orientation of the string. Describe the dependence of the trajectory of the stroller on system parameters. Assume both the mother and the stroller have negligible size. We recommend that you numerically simulate this problem.

How long will it take for a spherical stone of mass $m$ to reach the bottom of a pond $d$ meters deep if you throw it in from height $h?$ How will the answer change if the stone is „flat“ and not spherical?

Model of a rocket contains a motor whose power output is constant as long as it is provided with fuel. The initial mass of the fuel is $m_{p}$, the mass of an empty rocket is $m_{0}$ and the amount of fuel burned by the motor grows linearly with time. What is the maximum height the rocket can reach assuming the gravitational field to be homogeneous and the air resistance to be negligible?

Pepička has bought a light bulb, two switches and a wire. Help: her to design a circuit such that if you change the state of any of the switches the light bulb will also change its state (from on to off or reverse). After you find the solution try to generalize it to any number of switches.

A man wants to swim across a river which flows at a speed of 2 km/h. He is able to swim at a speed of 1 km/h. What is the optimal trajectory and direction he should take so that his trip is the least exhausting? Where and at what time will he reach the other bank? How would the situation change if his aim was the shortest possible trajectory? The width of the river is $d=10\;\mathrm{m}$.

• How would you draw (using only drawing-compass and a ruler) the Zhukovsky profil?
• Draw the streamlines around the Zhukovsky profile. Choose the parameters $d/l$ and $m/l$ so that they have real world justification.
• What is the lifting force acting on a square board? On a semicircular board?
• Draw the profile of a wing that corresponds to the Kármán–Trefftz transform.

• Convince yourselves that the nth roots of a complex number of modulus one lie on a regular $n-gon$ and solve the Bombelli equation $x^{3}-15x-4$ = 0. (see the text for hints)
• Express the identities concerning sin(α+β) and cos(α+β) using the complex exponential.
• Show that we were allowed to neglect the higher powers in deriving the Bernoulli limit, i.e. show that it was legitimate to add the o(1/$N)$ term inside the parentheses.
• Use the little-o notation to solve the problem of small oscillations around equilibrium point in Yukawa potential $V$ = $k \exp(x/λ) /$ $x$.
• Prove that the Chebyshev polynomials cos($n$ arccos $x)$ are really polynomials.

Hint: Let's have a unit complex number $z$ with real part $x$. Then, the expression is equal to the real part of $z^{n}$.

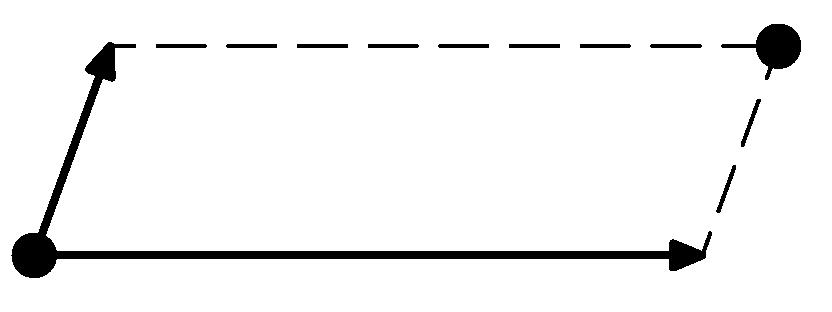
Choosing two different vectors in a plane and shifting the origin, an infinite grid of nodes can be created (see picture). Using the same approach in 3D will make a crystal lattice. If such grid is shifted by one of the vectors, we will get identical grid. Also rotation of the grid by some angle will generate identical grid. Find out all angles which can be basis for rotationaly symetrical grid and draw how such grid looks like.