Search

An object of mass $m$ on a piece of rubber of length $l_{0}is$ hung at a rigid point, the coordinates of which are $x=0$ and $y=0$. From the $xaxis$, which is horizontal, we slowly release the mass. What will be the relation between the lowest point reached and its position on the axis $x?$

Two ships are sailing against each other, the first one with a velocity $u_{1}=4\;\mathrm{m}\cdot \mathrm{s}^{-1}$ and the second with a velocity of $u_{2}=6\;\mathrm{m}\cdot \mathrm{s}^{-1}$. When they are seperated by $s_{0}=50\;\mathrm{km}$, a seagull launches from the first ship and flies towards the second one. He is flying against the wind, his speed is $v_{1}=20\;\mathrm{m}\cdot \mathrm{s}^{-1}$. When he arrives to the second ship he turns around and flies back now with the wind behind his back with a velocity $v_{2}=30\;\mathrm{m}\cdot \mathrm{s}^{-1}.He$ keps on flying back and forth until the two ships meet. How long is the path that he has undertaken?

A passanger on a ferry forgot to set the parking brake. Assume that the axis of the car is aligned with the axis of the ferry, and that because of waves the ferry is undergoing a harmonic motion, i.e. $φ(t)=Φ\sin\left(ωt)$. How far from the edge of the ferry can the passenger park the car without worrying about it falling into the sea? Assume that the maximal amplitude of oscillations is slowly increasing from zero to Φ.

In the fairy tale Mrazik, Ivan fought several bandits, stole their clubs, and threw them so high up into the sky that they did not fall back until half a year later. What is the altitude the clubs had to reach in order to stay in the air for so long? Make a first guess and then go on and improve it. Carefully analyze all the approximations you made and explain why are these estimates most likely wrong. Furthermore, explain why it makes no sense for the clubs to fall back at the same spot where Ivan threw them.

If you throw a small marble of diameter r from a very tall building, it appears to become smaller as it falls down. What is the time dependence of the apparent radius if, initially, it is at rest and a distance $x_{0}$ away from your eyes. Also assume that you are watching the marble directly from above at all times. Feel free to neglect any friction forces acting on the marble.

Imagine a bomb that is free-falling on a military base of your enemies. Although you threw the bomb off an airplane, you should assume that its motion is vertical (i.e. no horizontal component of its velocity relative to the surface). Because of air friction, the falling bomb emits a sound that propagates at the speed c = 340 m/s. What is the maximal impact velocity of the bomb in order for your enemies to hear it before it kills them?

Assume that the Earth is a sphere and the surface distance between Dresden and Vienna is approximately $d=370$ km. How much is the distance reduced if you decide to dig a tunnel between those two cities instead of walking. Neglect the different altitudes. Compare the tunnel distance with walking distance. For simplicity, you can approximate trigonometric functions as $$ \mathrm{sin} α ≈ α - α^{3}/6 \,,\\ \mathrm{cos} α ≈ 1 - α^{2}/2 \,,\\ \mathrm{tg} α ≈ α + α^{3}/3 \,, $$ where the angle is assumed to be given in radians.

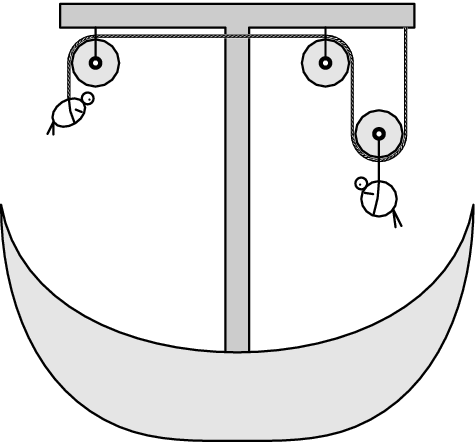
Two chubby residents of Venice Paolo and Francesca Muschetti (with masses $m_{P}=180\;\mathrm{kg}$ and $m_{F}=130\;\mathrm{kg})$ decided to go for a gondola ride. However, none of the gondoliers would allow them to enter the boat because it would sink. Fortunately, they managed to find one gondolier who designed the device shown on the picture. Both Paolo and Francesca were tied to the ends of the rope in such a way that at first Francesca was at the top but then she switched with Paolo. How tall should this device be in order for the boat to traverse the canal? The travel time is $τ=60\;\mathrm{s}$. Assume that if this device is used, the gondola does not sink. You can neglect any friction, the mass of the rope and the moments of inertia of all the pulleys.

Imagine the toy for hamsters depicted in the picture. The cylinder is free to rotate around the center point $O$. The hamster stands on the horizontal plate that is glued to the cylinder at a distance $h$ from the axis of rotation. How should the hamster move in order for the plate to stay in the horizontal position? The coefficient of friction between the hamster and the plate is $f$.

Two cars are driving towards each other with speed $v_{0}$. At what distance from each other should they hit the brakes in order to avoid a crash? Consider the case when both cars are on a flat road as well as the case when they are on a road inclined at an angle $α$. Both drivers apply the brakes at the same time. The braking force is equal to $f\cdot N$ where $N$ is the component of the car's weight normal to the road.